3025. Find the Number of Ways to Place People I

Problem Link

Description

---

You are given a 2D array points of size n x 2 representing integer coordinates of some points on a 2D plane, where points[i] = [xi, yi].

Count the number of pairs of points (A, B), where

• A is on the upper left side of B, and
• there are no other points in the rectangle (or line) they make (including the border).

Return the count.

 

Example 1:

Input: points = [[1,1],[2,2],[3,3]]

Output: 0

Explanation:

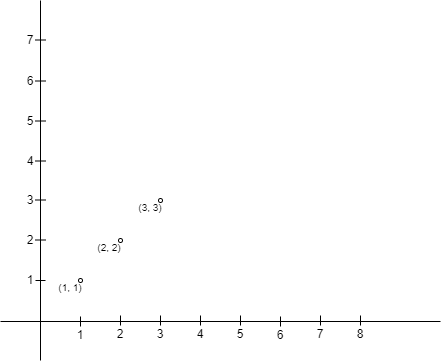
There is no way to choose A and B so A is on the upper left side of B.

Example 2:

Input: points = [[6,2],[4,4],[2,6]]

Output: 2

Explanation:

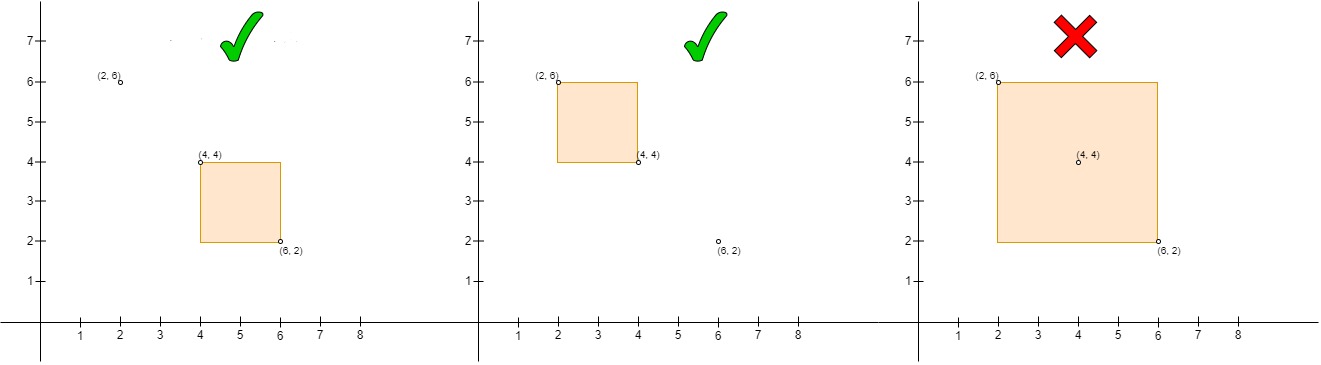
• The left one is the pair (points[1], points[0]), where points[1] is on the upper left side of points[0] and the rectangle is empty.
• The middle one is the pair (points[2], points[1]), same as the left one it is a valid pair.
• The right one is the pair (points[2], points[0]), where points[2] is on the upper left side of points[0], but points[1] is inside the rectangle so it's not a valid pair.

Example 3:

Input: points = [[3,1],[1,3],[1,1]]

Output: 2

Explanation:

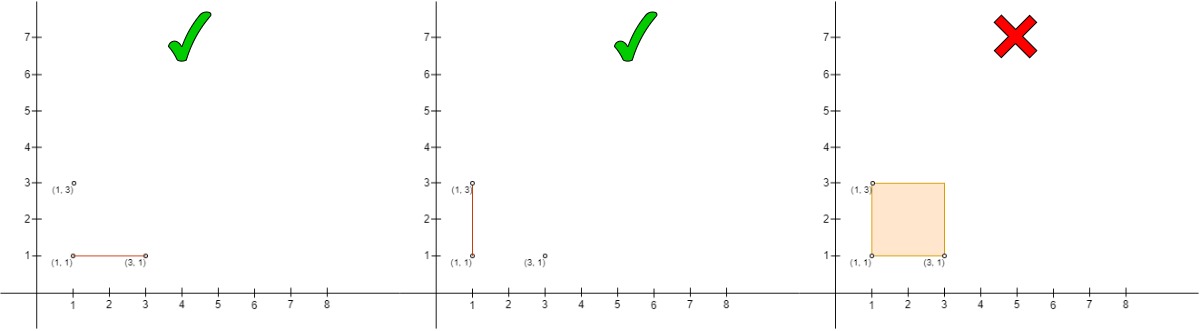
• The left one is the pair (points[2], points[0]), where points[2] is on the upper left side of points[0] and there are no other points on the line they form. Note that it is a valid state when the two points form a line.
• The middle one is the pair (points[1], points[2]), it is a valid pair same as the left one.
• The right one is the pair (points[1], points[0]), it is not a valid pair as points[2] is on the border of the rectangle.

 

Constraints:

• 2 <= n <= 50
• points[i].length == 2
• 0 <= points[i][0], points[i][1] <= 50
• All points[i] are distinct.

Solution

---

Python3

class Solution:
    def numberOfPairs(self, points: List[List[int]]) -> int:
        N = len(points)
        res = 0
        points.sort(key = lambda x : (x[0], -x[1]))
 
        for i in range(N):
            x1, y1 = points[i]
            y = -inf
            for j in range(i + 1, N):
                x2, y2 = points[j]
                # x1 <= x2
 
                if y1 >= y2 and y2 > y:
                    res += 1
                    y = y2
        
        return res